if $150 grows to $240 in n years, what will $1000 grow to over the same period

This compound involvement calculator is a tool to assist you estimate how much money yous will earn on your deposit. In gild to brand smart fiscal decisions, y'all need to exist able to foresee the final outcome. That's why it's worth knowing how to calculate compound interest. The nigh common real-life application of the compound interest formula is a regular savings calculation.

Read on to find answers to the following questions:

• What is the interest charge per unit definition?
• What is the compound interest definition and what is the compound interest formula?
• What is a difference betwixt unproblematic and compound interest rates?
• How to calculate compound involvement?
• What are the nigh mutual compounding frequencies?

Y'all may as well want to check our educatee loan reckoner where yous can make a projection on your expenses and study the upshot of dissimilar educatee loan options on your upkeep.

Interest rate definition

In finance, involvement charge per unit is divers as the amount charged by a lender to a borrower for the utilise of an asset. And then, for the borrower the involvement rate is the cost of the debt, while for the lender information technology is the rate of return.

Note that in the case where y'all make a eolith into a depository financial institution (e.g., put money in your savings account), you lot have, from a financial perspective, lent money to the depository financial institution. In such a case the involvement rate reflects your profit.

The interest rate is commonly expressed equally a percentage of the main corporeality (outstanding loan or value of deposit). Normally, it is presented on an annual basis, which is known as the almanac percentage yield (APY) or constructive annual rate (EAR).

What is the compound interest definition?

More often than not, compound interest is defined equally involvement that is earned not solely on the initial amount invested but as well on any further interest. In other words, compound interest is the involvement on both the initial principal and the involvement which has been accumulated on this principle so far. Therefore, the key characteristic of compound interest is that interest itself earns interest. This concept of adding a carrying charge makes a eolith or loan grow at a faster rate.

You lot can utilize the compound interest equation to discover the value of an investment afterwards a specified period or estimate the charge per unit you accept earned when buying and selling some investments. It also allows you to answer some other questions, such every bit how long it will have to double your investment.

We will answer these questions in the examples below.

Simple vs. compound interest

You lot should know that unproblematic interest is something different than the compound involvement. It is calculated only on the initial sum of money. On the other hand, compound interest is the interest on the initial principal plus the interest which has been accumulated.

Compounding frequency

Well-nigh fiscal advisors will tell you that the compound frequency is the compounding periods in a yr. But if you are non certain what compounding is, this definition will be meaningless to you… To empathise this term y'all should know that compounding frequency is an answer to the question How frequently is the interest added to the principal each year? In other words, compounding frequency is the time period after which the interest volition be calculated on top of the initial amount.

For example:

• annual (1/Year) compounding has a compounding frequency of one,
• quarterly (4/Year) compounding has a compounding frequency of four,
• monthly (12/Yr) compounding has a compounding frequency of twelve.

Notation that the greater the compounding frequency is, the greater the last balance. However, even when the frequency is unusually high, the terminal value tin can't rise to a higher place a particular limit. To empathize the math backside this, bank check out our natural logarithm calculator.

As the principal focus of the calculator is the compounding mechanism, nosotros designed a nautical chart where you can follow the progress of the annual interest balances visually. If you cull a higher than yearly compounding frequency, the diagram will brandish the resulting actress or additional role of involvement gained over yearly compounding by the higher frequency. Thus, in this mode, you can easily find the existent ability of compounding.

Compound interest formula

The chemical compound involvement formula is an equation that lets y'all gauge how much you will earn with your savings account. It's quite circuitous considering information technology takes into consideration non merely the annual interest charge per unit and the number of years but also the number of times the interest is compounded per year.

The formula for annual compound interest is as follows:

FV = P (1+ r/m)^mt

Where:

• FV - the future value of the investment, in our calculator it is the last balance
• P - the initial remainder (the value of the investment)
• r - the almanac interest rate (in decimal)
• m - the number of times the interest is compounded per year (compounding frequency)
• t - the numbers of years the coin is invested for

It is worth knowing that when the compounding period is 1 (m = one) then the interest charge per unit (r) is telephone call the CAGR (compound annual growth rate).

How to calculate compound interest

Really, yous don't need to memorize the chemical compound involvement formula from the previous department to approximate the time to come value of your investment. In fact, y'all don't even need to know how to calculate chemical compound interest! Cheers to our compound interest calculator you tin can exercise it in merely a few seconds, whenever and wherever y'all desire. (NB: Have yous already tried the mobile version of our calculators?)

With our smart calculator, all you need to calculate the future value of your investment is to fill the advisable fields:

• Main properties

1. Initial balance - the amount of coin you are going to invest or deposit.
2. Interest rate – the involvement charge per unit expressed on a yearly basis.
3. Term – the fourth dimension frame you are going to invest money.
4. Compound frequency – in this field, you should select how often the compounding applies to your balance. Usually, the interest added to the principal residuum daily, weekly, monthly, quarterly, semi-annually, or yearly. Only yous may set it as continuous compounding as well, which is the theoretical limit for the compounding frequency. In this example, the number of periods when compounding occurs is infinite.

• Additional deposits

1. How much - the corporeality y'all are planning to deposit on the account.
2. How often - you can cull the frequency of the boosted deposit here.
3. When - you should select the timing of the transaction of the additional deposit. More specifically, you may place the money to the business relationship at the beginning or at the end of the periods.
4. Growth charge per unit of deposit - this option allows you to set a growth rate of the additional eolith. This choice can be particularly useful in the long term when your income possibly increases due, for example, to inflation and/or promotions.

That's information technology! In a flash, our compound involvement calculator makes all necessary computations for you and gives you the results.

The two main results are:

• the terminal balance, that is the total corporeality of money you volition receive later on the specified menstruum, and
• the full involvement, which is the total compounded involvement payment.

In instance you set the boosted deposit field, we gave you the results for the compounded initial residue and compounded boosted remainder.

Likewise, we besides show you their contribution to the full involvement corporeality, namely, interest on the initial balance and interest on the additional deposit.

Compound interest examples

• Do you desire to understand the compound interest equation?
• Are yous curious about the fine details of how to calculate the chemical compound interest rate?
• Are you wondering how our calculator works?
• Do y'all need to know how to interpret the results of compound involvement calculation?
• Are you lot interested in all possible uses of the chemical compound involvement formula?

The following examples are there to effort and help you answer these questions. We believe that after studying them, yous won't have any trouble with the understanding and practical implementation of compound interest.

Example 1 – basic calculation of the value of an investment

The kickoff example is the simplest, in which we summate the future value of an initial investment.

Question

You lot invest $10,000 for ten years at the annual interest rate of five%. The interest rate is compounded yearly. What will be the value of your investment after x years?

Solution

Firstly let's determine what values are given, and what we demand to find. Nosotros know that you are going to invest $10,000 - this is your initial residuum P, and the number of years you are going to invest money is 10. Moreover, the interest charge per unit r is equal to 5%, and the interest is compounded on a yearly footing, so the m in the compound interest formula is equal to 1.

We want to calculate the amount of money you will receive from this investment, that is, we want to detect the future value FV of your investment.

To count it, we need to plug in the appropriate numbers into the compound interest formula:

FV = 10,000 * (1 + 0.05/1) ^ (ten*1) = 10,000 * 1.628895 = 16,288.95

Reply

The value of your investment after ten years will be $sixteen,288.95.

Your turn a profit will be FV - P. It is $sixteen,288.95 - $ten,000.00 = $6,288.95.

Note that when doing calculations you must be very careful with your rounding. You shouldn't do too much until the very end. Otherwise, your respond may be wrong. The accuracy is dependent on the values you are computing. For standard calculations, half dozen digits afterward the decimal betoken should be enough.

Example two - complex calculation of the value of an investment

In the second example, nosotros summate the futurity value of an initial investment in which interest is compounded monthly.

Question

You invest $10,000 at the annual interest rate of 5%. The interest rate is compounded monthly. What will exist the value of your investment afterwards 10 years?

Solution

Like in the first example, we should determine the values first. The initial balance P is $10,000, the number of years y'all are going to invest money is 10, the involvement rate r is equal to 5%, and the compounding frequency thou is 12. We need to obtain the future value FV of the investment.

Allow'due south plug in the advisable numbers in the compound interest formula:

FV = 10,000 * (1 + 0.05/12) ^ (x*12) = 10,000 * ane.004167 ^ 120 = ten,000 * 1.647009 = 16,470.09

Answer

The value of your investment afterwards 10 years will exist $16,470.09.

Your turn a profit will be FV - P. Information technology is $sixteen,470.09 - $10,000.00 = $vi,470.09.

Did yous notice that this case is quite like to the first one? Actually, the merely difference is the compounding frequency. Note that, simply thank you to more frequent compounding this fourth dimension you volition earn $181.14 more during the same period! ($vi,470.09 - $six,288.95 = $181.xiv)

Example iii - Calculating the involvement rate of an investment using the compound interest formula

Now, let'due south endeavour a different type of question that tin can be answered using the chemical compound interest formula. This time, some basic algebra transformations will be required. In this example, we will consider a situation in which nosotros know the initial balance, final residual, number of years and compounding frequency but nosotros are asked to summate the interest charge per unit. This blazon of calculation may be applied in a state of affairs where yous want to determine the rate earned when buying and selling an asset (due east.k., property) which you are using as an investment.

Data and question
Yous bought an original painting for $2,000. Six years after, yous sold this painting for $3,000. Assuming that the painting is viewed as an investment, what annual rate did you lot earn?

Solution
Firstly, let's decide the given values. The initial residuum P is $ii,000 and final balance FV is $3,000. The time horizon of the investment vi years and the frequency of the calculating is 1. This fourth dimension, we need to compute the involvement rate r.

Let's endeavor to plug this numbers in the basic compound involvement formula:

3,000 = 2,000 * (1 + r/one) ^ (6*i)

So:

3,000 = 2,000 * (1 + r) ^ (6)

We can solve this equation using the following steps:
Dissever both sides by 2000

three,000 / 2,000= (i + r) ^ (6)

Raise both sides to the 1/6th power

(3,000 / two,000) ^ (one / 6) = (1 + r)

Subtract ane from both sides

(three,000 / 2,000) ^ (1 / six) – 1 = r

Finally solve for r

r = ane.5 ^ 0.166667 – i = ane.069913 - one = 0.069913 = six.9913%

Reply

In this example you lot earned $1,000 out of the initial investment of $ii,000 within the half-dozen years, meaning that your annual rate was equal to 6.9913%.

As yous can see this fourth dimension, the formula is not very elementary and requires a lot of calculations. That's why it'south worth testing our compound involvement calculator, which solves the same equations in an instant, saving you lot time and effort.

Example four - Calculating the doubling time of an investment using the compound interest formula

Have you ever wondered how many years information technology will take for your investment to double its value? Likewise its other capabilities, our reckoner tin can help you to respond this question. To understand how it does it, let's take a await at the following example.

Data and question

You put $1,000 on your saving account. Bold that the interest rate is equal to 4% and it is compounded yearly. Find the number of years after which the initial balance will double.

Solution

The given values are equally follows: the initial balance P is $1,000 and final balance FV is ii * $i,000 = $2,000, and the interest rate r is 4%. The frequency of the computing is i. The time horizon of the investment t is unknown.

Permit's start with the bones compound interest equation:

FV = P (1 + r/k)^mt

Knowing that m = one, r = 4%, and 'FV = 2 * P we can write

2P = P (one + 0.04) ^ t

Which could be written as

2P = P (1.04) ^ t

Divide both sides by P (P mustn't be 0!)

ii = 1.04 ^ t

To solve for t, y'all need take the natural log (ln), of both sides:

ln(ii) = t * ln(1.04)

And so

t = ln(two) / ln(one.04) = 0.693147 / 0.039221 = 17.67

Answer

In our example it takes 18 years (eighteen is the nearest integer that is higher than 17.67) to double the initial investment.

Have you noticed that in the above solution we didn't even need to know the initial and final balances of the investment? It is cheers to the simplification we made in the 3rd step (Divide both sides by P). However, when using our compound interest charge per unit calculator, you will need to provide this information in the appropriate fields. Don't worry if you just want to find the time in which the given interest charge per unit would double your investment, just blazon in any numbers (for instance 1 and 2).

It is also worth knowing that exactly the aforementioned calculations may exist used to compute when the investment would triple (or multiply past any number in fact). All y'all need to do is merely use a different multiple of P in the 2nd step of the above instance. You tin can also do it with our calculator.

Compound involvement table

Compound involvement tables were used everyday, earlier the era of calculators, personal computers, spreadsheets, and unbelievable solutions provided past Omni Calculator 😂. The tables were designed to make the financial calculations simpler and faster (yes, really…). They are included in many older financial textbooks as an appendix.

Beneath, you tin see what a compound involvement tabular array looks like.

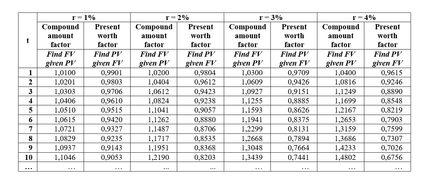
Using the information provided in the compound interest table y'all can calculate the last residue of your investment. All you need to know is that the column compound amount gene shows the value of the factor (ane + r)^t for the respective interest charge per unit (starting time row) and t (get-go column). So to calculate the final residue of the investment you demand to multiply the initial balance by the appropriate value from the table.

Note that the values from the column Present worth cistron are used to compute the present value of the investment when you know its future value.

Obviously, this is merely a basic example of a compound interest table. In fact, they are usually much, much larger, as they incorporate more periods t diverse interest rates r and different compounding frequencies m... You had to flip through dozens of pages to find the appropriate value of compound amount factor or present worth factor.

With your new knowledge of how the world of financial calculations looked before Omni Calculator, do you savor our tool? Why not share it with your friends? Permit them know about Omni! If you want to be financially smart, you can too try our other finance calculators.

Boosted Information

Now that you know how to calculate compound involvement, it'due south high time you plant other applications to help you make the greatest turn a profit from your investments:

To compare banking company offers which have different compounding periods, we need to summate the Almanac Percentage Yield, also called Constructive Almanac Rate (EAR). This value tells the states how much profit nosotros will earn within a year. The well-nigh comfy fashion to effigy it out is using the APY computer, which estimates the EAR from the involvement rate and compounding frequency.

If yous want to detect out how long information technology would have for something to increment by n%, you can use our rule of 72 estimator. This tool enables you to cheque how much time you need to double your investment fifty-fifty quicker than the compound involvement charge per unit computer.

You lot may too be interested in the credit card payoff figurer, which allows you to estimate how long information technology will take until you are completely debt-complimentary.

Another interesting calculator is our cap charge per unit calculator which determines the rate of return on your real estate holding purchase.

Nosotros also suggest you try the lease estimator which helps you decide the monthly and total payments for a lease.

If you're looking to finance the purchase of a new recreational vehicle (RV), our RV loan figurer makes it unproblematic to work out what the best bargain will be for you.

The depreciation figurer enables you to apply three dissimilar methods to estimate how fast the value of your asset decreases over time.

And finally, why not to try our dream come true calculator.
which answers the question: how long exercise you have to relieve to afford your dream?

stewartphim1981.blogspot.com

Source: https://www.omnicalculator.com/finance/compound-interest

Share this post